Written by: Paula Clark
May 27, 2022
Leather goods, artisan cheeses, and the perfect pair of jeans. These are just some of the things that are known to improve with time. Unfortunately, lists of things that get better with time might fail to include mathematics when in reality, mathematics and number sense should top the list.
While it might make sense that cognition in any domain improves with the passage of time, the phrase getting better with time has an ulterior meaning. In its most basic form, time is a sensory experience. Natural time is our awareness of periodic and rhythmic changes in the world around us. Conversely, artificial time requires a shift in our cognitive functioning as our brains from mental representations of periodic occurrences. During this shift in cognitive functioning, as our brains transition from concrete to abstract thinking, mathematical content knowledge improves. Literally, we get better with time.
It’s in our DNA.
The passage of time is a universal experience. We mark the passage of time with annual birthday and holiday celebrations. We partition our day into intervals for work, play, and rest. We experience impatience when time moves too slowly or anxiety when time moves too quickly. Even infants have remarkable capabilities to understand the rhythm of time. This ability to sense natural time is not only rooted in our physiology, and it is strengthened by movement and experience.
But what about time as it relates to mathematics outcomes? To answer this question, we must consider how conceptualizing time is vastly different than telling time. Conceptualizing time involves grappling with magnitude, space, and orientation. It involves gathering information from our suppressing and excitement neurological processes. It involves using our senses to construct, test, and revise personal mental representations. Just as our ability to add, subtract, or compare numbers relates to our innate, mental number line, our ability to judge magnitude and proportionality relates to our inner clock.
Invisible and Untouchable
While the construct of time might very well be invisible and untouchable, analog clocks provide us with a concrete representation of time and its passing (Earnest & Chandler, 2019). By manipulating the hands of an analog clock, learners explore part to whole relationships, experience and compare varying units of time, develop proportional reasoning, and strengthen their temporal and spatial orientation. As with most manipulatives, the analog clock becomes a tool of engagement for students to use as they construct mental representations.
As classroom teachers and educational therapists, we have all encountered students who struggle with conceptual understandings, even when they can perform procedures from memory. Like time, students’ conceptual understandings of mathematics are often invisible. We have all worked with students who have no trouble telling time. They have perfected the art of memorizing procedures and can accurately relay the time to us. However, when we vary our presentation method or ask students to solve elapsed time problems, their lack of a conceptual understanding is made visible. The varying units (e.g., seconds, minutes, hours), displays (e.g., digital and analog), and language (e.g.. forty-five minutes after the hour and a quarter ‘till) pose unique problems for learners with dyslexia, dyscalculia, or processing differences. Having students reference an analog clock when performing tasks reduces the cognitive load and assists students in constructing meaning. By manipulating the hands on an analog clock, students strengthen their understanding of the time that has passed compared with the time remaining. In addition, the physical and visual manipulation provides them with a basis for understanding intervals as they relate to the whole.
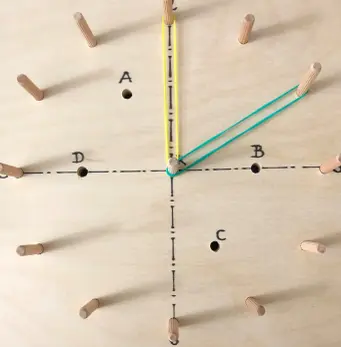
The GeoClock
I love finding educational tools whose function spans mathematics concepts and developmental levels. Such tools remind me of how mathematical knowledge is networked rather than linear. These tools also remind me that mathematical knowledge is a developmental process mediated by action. As an educator, I find that tools are more functional when they afford students a hands-on opportunity to experience rather than observe mathematics. Cognitive neuroscientist Vincent Walsh (2015) reiterates that which educators know: “action is the beginning of all cognition” (p. 561). Therefore, tools that require students to be active learners and constructors of knowledge are high on my list.
I think I found such a tool in the GeoClock. The GeoClock educational tool, developed by Nikosz and Agnes Jordanidisz, is a mathematical resource that bridges action and cognition. The GeoClock method aims to develop students’ temporal and spatial orientation while enhancing their understanding of fractions, angles, and geometric shapes. Foundational to the GeoClock method is the reference to time. In fact, fundamental lessons and therapy sessions begin with assisting students with visualizing and manipulating time. Students quickly progress and intuitively make connections between time, angles, fractions, and geometric shapes. More than that, students develop the cognitive flexibility necessary to transfer and apply these basic mathematical skills across domains. Using the GeoClock, students become fluent in describing 3 o’clock as a quarter of an hour and the space between these hands on a circular clock as a fourth of a circle. In the 3 o’clock position, the clock hands become rays of an angle that intersect perpendicularly. Students naturally derive that perpendicular lines form 90-degree or right angles.
Having taught mathematics across all grade levels, I appreciate how the GeoClock develops students’ capacity for learning along with their mathematics content knowledge. When engaging with the GeoClock, students reason and problem-solve. They construct and critique arguments, and they take notice of patterns. Ultimately, students are actively engaged in refining their innate mathematical abilities. In The Number Sense, Dehaene (2011) suggests that students often encounter difficulties in mathematics because in-school mathematics vastly differs from the mathematics of nature. The GeoClock bridges these worlds by assisting students in developing and strengthening their innate abilities.
The Importance of Language
I was recently introduced to the book Teaching for the Students: Habits of Heart, Mind, and Practice in the Engaged Classroom by Bob Fecho. I was immediately drawn to Fecho’s description of learning: “learning is a generative act, one in which inquiry, critique, dialogue shape[s] learners” (Fecho, 2011, p. 9). I immediately became fixated on the word dialogue in this definition. After all, it is our ability to use language to communicate and to engage in dialogue with others that allows us to refine our cognition.
More than a static manipulative, the GeoClock is a dynamic, dialogical tool that mediates reasoning and problem-solving in reference to time, fractions, and proportional understanding. When used in educational therapy, the GeoClock develops students’ cognitive flexibility and assists them in applying mathematics concepts across domains.
Paula Clark, Ed.D.
References
Dehaene, S. (2020). How we learn: The new science of education and the brain. Penguin UK.
Dehaene, S. (2011). The number sense: How the mind creates mathematics. OUP USA.
Earnest, D., & Chandler, J. (2019). Time Expressions and Elementary Students' Reasoning with the Analog Clock. North American Chapter of the International Group for the Psychology of Mathematics Education.
Fecho, B. (2011). Teaching for the students: Habits of heart, mind, and practice in the engaged classroom. Teachers College Press.
Walsh, V. (2015). A theory of magnitude: The parts that sum to number. In R. C. Kadosh & A. Dowker (Eds.), The Oxford handbook of numerical cognition (pp. 552–565). Oxford University Press.